抱着负责的心态把要讲的东西重写了一遍。
[TOC]
在计算几何题中,各种图形的表示往往是以坐标形式出现的,因此我们需要对这些坐标进行一个好的存储,从而方便我们进行操作。
我们有时会遇见精度问题,于是整个好玩的判一下就行了。
1 2 3 4 5 6 7 const double eps = 1e-8 ;int ceps (double x) if (fabs (x) < eps) return 0 ; if (x < 0 ) return -1 ; return 1 ; }
这个十分简单,一个点只有 x x x y y y
另一种好的方式是 pair。
1 2 3 4 5 6 7 struct poi //点或向量{ double x,y; poi (double X = 0 , double Y = 0 ){x = X, y = Y;} }; typedef poi vecpair<double ,double > poi;
任意两点之间 P 1 ( x 1 , y 1 ) , P 2 ( x 2 , y 2 ) P_1(x_1,y_1),P_2(x_2,y_2) P 1 ( x 1 , y 1 ) , P 2 ( x 2 , y 2 )
∣ P 1 P 2 ∣ = ( x 2 − x 1 ) 2 + ( y 2 − y 1 ) 2 |P_1P_2| = \sqrt{(x_2-x_1)^2 + (y_2-y_1)^2} ∣ P 1 P 2 ∣ = ( x 2 − x 1 ) 2 + ( y 2 − y 1 ) 2
1 2 3 4 double dis (poi a,poi b) return (double ) sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)) }
两点确定一条直线,所以我们存两个点。
另一种表示,可以是一个点加上一个方向向量。
但是这里只写点表示法了。
1 2 3 4 5 struct line { poi A,B; line (poi a,poi b){A = a, B = b;} }
同样道理,左右端点就ok。
1 2 3 4 5 struct Segment { poi A,B; Segment (poi a,poi b) {A = a, B = b;} }
考虑一下,emm,多边形不就是一堆点嘛。。。
思考,坐标系上的一个圆…
很显然会有圆心和半径,那么存个圆心存个半径就行了。
1 2 3 4 5 6 struct circle { poi p; double r; circle (point A,double B){p = A, r = B;} }
至此,表示方法就结束了。
等等,是不是有另一种表示方法?
有的!
选一个 O O O 1 1 1
IG要无了,我shy哥还没上场
我们先在这里放一张图:
在极坐标系中,设 A A A O O O A A A ∣ O A ∣ |OA| ∣ O A ∣ ρ \rho ρ O A OA O A ∠ x O A \angle xOA ∠ x O A β \beta β ( ρ , β ) (\rho,\beta) ( ρ , β ) A A A
有些时候我们需要把极坐标系转化到平面直角坐标系下去研究,两者转化关系如下:
点 A ( ρ , θ ) A(\rho,\theta) A ( ρ , θ ) ( x , y ) (x,y) ( x , y ) { ρ cos θ = x ρ sin θ = y \left\{\begin{aligned} \rho \cos\theta&=x\\\rho\sin\theta&=y\end{aligned}\right. { ρ cos θ ρ sin θ = x = y
进而可知: { ρ 2 = x 2 + y 2 tan θ = y x \left\{\begin{aligned} \rho^2 &=x^2 + y^2\\\tan\theta&={y\over x}\end{aligned}\right. ⎩ ⎨ ⎧ ρ 2 tan θ = x 2 + y 2 = x y
于是极角 $\theta = \arctan{y\over x} $ ,然后这样就可以求出极角了。
如果题目中要求反正切函数,尽量使用 a t a n 2 ( y , x ) atan2({y,x}) a t a n 2 ( y , x ) a t a n ( x ) atan(x) a t a n ( x )
搬了搬了
sin ( a + θ ) = sin a cos θ + cos a sin θ \sin(a + \theta) = \sin a\cos \theta + \cos a\sin \theta sin ( a + θ ) = sin a cos θ + cos a sin θ
sin ( a − θ ) = sin a cos θ − cos a sin θ \sin(a-\theta) = \sin a\cos \theta - \cos a\sin \theta sin ( a − θ ) = sin a cos θ − cos a sin θ
cos ( a + θ ) = cos a cos θ − sin a sin θ \cos(a + \theta) = \cos a\cos \theta - \sin a\sin \theta cos ( a + θ ) = cos a cos θ − sin a sin θ
cos ( a − θ ) = cos a cos θ + sin a sin θ \cos(a - \theta) = \cos a \cos \theta + \sin a\sin \theta cos ( a − θ ) = cos a cos θ + sin a sin θ
矢量就是向量…
若向量 a ⃗ = ( x , y ) \vec a = (x,y) a = ( x , y ) ∣ a ∣ = x 2 + y 2 = ∣ a ⃗ ∣ 2 |a| = \sqrt{x^2+y^2} = \sqrt{|\vec a|^2} ∣ a ∣ = x 2 + y 2 = ∣ a ∣ 2
1 2 3 4 double len (poi a) return (double ) sqrt (a.x*a.x+a.y*a.y); }
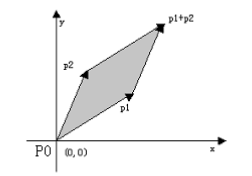
对于 a ⃗ = ( x 1 , y 1 ) \vec a = (x_1,y_1) a = ( x 1 , y 1 ) b ⃗ = ( x 2 , y 2 ) \vec b = (x_2,y_2) b = ( x 2 , y 2 ) a ⃗ + b ⃗ = ( x 1 + x 2 , y 1 + y 2 ) \vec a + \vec b = (x_1+x_2,y_1+y_2) a + b = ( x 1 + x 2 , y 1 + y 2 )
对于 a ⃗ = ( x 1 , y 1 ) \vec a = (x_1,y_1) a = ( x 1 , y 1 ) b ⃗ = ( x 2 , y 2 ) \vec b = (x_2,y_2) b = ( x 2 , y 2 ) a ⃗ − b ⃗ = ( x 1 − x 2 , y 1 − y 2 ) \vec a -\vec b = (x_1-x_2,y_1-y_2) a − b = ( x 1 − x 2 , y 1 − y 2 )
1 2 poi operator + (poi a,poi b) {return poi (a.x+b.x,a.y+b.y);} poi operator - (poi a,poi b) {return poi (a.x-b.x,a.y-b.y);}
对于 $\vec a = (x,y) $ , λ a ⃗ = ( λ x , λ y ) \lambda\vec a = (\lambda x,\lambda y) λ a = ( λ x , λ y )
1 poi operator * (poi a,double k) {return poi (a.x*k,a.y*k);}
数量积也就是点积,结果是一个数,大小等于这两个矢量的模长的乘积,再乘以他们夹角的余弦,即: a ⋅ b = ∣ a ∣ ∣ b ∣ cos < a , b > a \cdot b = |a||b|\cos<a,b> a ⋅ b = ∣ a ∣ ∣ b ∣ cos < a , b >
对于 a ⃗ = ( x 1 , y 1 ) \vec a = (x_1,y_1) a = ( x 1 , y 1 ) b ⃗ = ( x 2 , y 2 ) \vec b = (x_2,y_2) b = ( x 2 , y 2 ) a ⃗ ⋅ b ⃗ = ( x 1 x 2 + y 1 y 2 ) \vec a \cdot \vec b = (x_1x_2+y_1y_2) a ⋅ b = ( x 1 x 2 + y 1 y 2 )
夹角 θ \theta θ
若 θ = 0 \theta = 0 θ = 0 a ⃗ ⋅ b ⃗ = ∣ a ∣ ∣ b ∣ \vec a\cdot \vec b = |a||b| a ⋅ b = ∣ a ∣ ∣ b ∣
若 θ = 18 0 ∘ \theta = 180^\circ θ = 1 8 0 ∘ a ⃗ ⋅ b ⃗ = − ∣ a ∣ ∣ b ∣ \vec a \cdot \vec b = -|a||b| a ⋅ b = − ∣ a ∣ ∣ b ∣
若 θ < 9 0 ∘ \theta < 90^\circ θ < 9 0 ∘ a ⃗ ⋅ b ⃗ > 0 \vec a\cdot \vec b > 0 a ⋅ b > 0
若 θ = 9 0 ∘ \theta = 90^\circ θ = 9 0 ∘ a ⃗ ⋅ b ⃗ = 0 \vec a \cdot \vec b = 0 a ⋅ b = 0
若 θ > 9 0 ∘ \theta > 90^\circ θ > 9 0 ∘ a ⃗ ⋅ b ⃗ < 0 \vec a\cdot\vec b < 0 a ⋅ b < 0
1 double Dot (poi a,poi b) return a.x*b.x+a.y*b.y;}
满足以下几个性质:
交换律: a ⃗ ⋅ b ⃗ = b ⃗ ⋅ a ⃗ \vec a \cdot \vec b = \vec b\cdot \vec a a ⋅ b = b ⋅ a
分配律: a ⃗ ⋅ ( b ⃗ + c ⃗ ) = a ⃗ ⋅ b ⃗ + a ⃗ ⋅ c ⃗ \vec a\cdot (\vec b + \vec c) = \vec a\cdot \vec b + \vec a \cdot \vec c a ⋅ ( b + c ) = a ⋅ b + a ⋅ c
结合律: ( m a ⃗ ) ⋅ b ⃗ = m ( a ⃗ ⋅ b ⃗ ) = a ⃗ ⋅ ( m b ⃗ ) (m\vec a) \cdot \vec b = m (\vec a\cdot \vec b) = \vec a \cdot (m\vec b) ( m a ) ⋅ b = m ( a ⋅ b ) = a ⋅ ( m b )
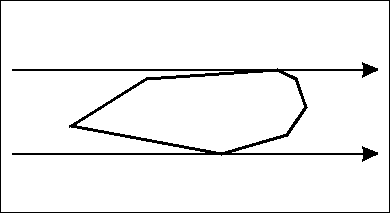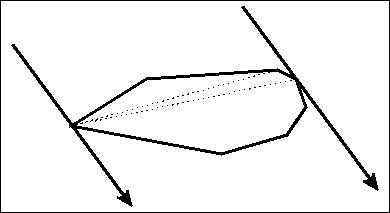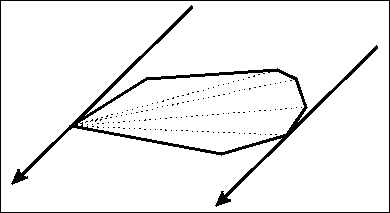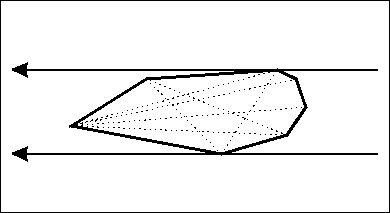
也叫叉积,其结果是一个向量。
矢量 a a a b b b a a a b b b ∣ a ⃗ × b ⃗ ∣ = ∣ a ⃗ ∣ ∣ b ⃗ ∣ sin < a , b > |\vec a \times \vec b| = |\vec a||\vec b| \sin<a,b> ∣ a × b ∣ = ∣ a ∣ ∣ b ∣ sin < a , b >
对于 a ⃗ = ( x 1 , y 1 ) \vec a = (x_1,y_1 ) a = ( x 1 , y 1 ) b ⃗ = ( x 2 , y 2 ) \vec b = (x_2,y_2) b = ( x 2 , y 2 ) a ⃗ × b ⃗ = ( x 1 y 2 − x 2 y 1 ) \vec a\times \vec b = (x_1y_2-x_2y_1) a × b = ( x 1 y 2 − x 2 y 1 )
如图所示,向量位置与叉积模长大小的关系:
若 a ⃗ × b ⃗ = 0 \vec a\times \vec b = 0 a × b = 0 a ⃗ \vec a a b ⃗ \vec b b
若 a ⃗ × b ⃗ > 0 \vec a\times \vec b > 0 a × b > 0 a ⃗ \vec a a b ⃗ \vec b b
若 a ⃗ × b ⃗ < 0 \vec a\times \vec b < 0 a × b < 0 a ⃗ \vec a a b ⃗ \vec b b
1 double Cro (poi a,poi b) return a.x*b.y-a.y*b.x;}
注意 sin \sin sin a ⃗ \vec a a b ⃗ \vec b b sin < a ⃗ , b ⃗ > \sin<\vec a,\vec b> sin < a , b >
又因为前面乘的是绝对值,所以叉积的符号就是 sin \sin sin
三角形面积公式:2 S Δ A B C = a b sin C 2S_{\Delta ABC} = ab\sin C 2 S Δ A B C = a b sin C
因此,三角形的面积就是任意两条临边的叉积绝对值的一半。
同理,平行四边行的面积就是两条临边对应向量的叉积的绝对值。
类似的,任意四边形的面积等于两条对角线的叉积的绝对值的一半。
v ′ = ( x cos a − y sin a , x sin a + y cos a ) v^\prime = (x\cos a - y\sin a, x\sin a + y\cos a) v ′ = ( x cos a − y sin a , x sin a + y cos a )
其中 a a a
如果实在记不住,就把直角坐标转化为极坐标,在把角度加上 a a a
1 2 3 4 poi rtt (poi a,double altha) return poi (a.x * cos (altha) - a.y * sin (altha),a.x * sin (altha) + a.y *cos (altha)); }
例题: uva11178
做法1: 点 p p p A B AB A B ∣ P A ∣ + ∣ P B ∣ = ∣ A B ∣ |PA| + |PB| = |AB| ∣ P A ∣ + ∣ P B ∣ = ∣ A B ∣
做法2:先利用叉积判断点是否在线段所在的直线上(叉积为 0),在用点积判断点是否在线段上(点积小于等于 0) 即可。如果点在线段的端点上点积等于零,否则点积小于零。
一般来说,做法 1 1 1
1 2 3 4 5 bool pd_PS (poi p,poi A,poi B) return (ceps (Cro (p-A,p-B)) == 0 ) &&(ceps (Dot (p-A,p-B) <= 0 )); }
口胡:如果说 p p p A B A B A B A P ⃗ \vec {AP} A P B P ⃗ \vec {BP} B P
从 P P P A B AB A B Q Q Q
若 Q Q Q A B AB A B P P P A B AB A B P Q PQ P Q
如果 Q Q Q B A BA B A P A PA P A P B PB P B
如果 ∠ P A B \angle PAB ∠ P A B ∠ P B A \angle PBA ∠ P B A Q Q Q A B AB A B ∠ P A B \angle PAB ∠ P A B Q Q Q B A BA B A ∠ P B A \angle PBA ∠ P B A Q Q Q A B AB A B
可以自己画一下图理解一下。
1 2 3 4 5 6 double dis_PS (point p,point A,point B) if (Dot (p-A,B-A) < 0 ) return dis (p,A); if (Dot (p-B,A-B) < 0 ) return dis (p,B); return abs (Cro (A-p,B-p))/dis (A,B); }
例题: UVA10263 Railway
如果两个线段相交,则必然有一条线段的两个端点在另一条线段的两侧。
利用叉积判断即可。
1 2 3 4 5 6 bool pd_SS (point A,point B,point C,point D) double f1 = Cro (C-A,B-A), f2 = Cro (D-A,B-A); double g1 = Cro (A-C,D-C), g2 = Cro (B-C,D-C); return ((f1 < 0 ) ^ (f2 < 0 )) && ((g1 < 0 ) ^ (g2 < 0 )); }
如图所示我们要求点 A A A B C BC B C
我们连接 A B AB A B A C AC A C A A A B C BC B C D D D
则有 2 S Δ A B C = A D ∗ B C 2S_{\Delta ABC} = AD * BC 2 S Δ A B C = A D ∗ B C
点 A A A A D AD A D 2 S Δ A B C B C 2S_{\Delta ABC} \over BC B C 2 S Δ A B C A D = ∣ A B ⃗ × A C ⃗ ∣ ∣ B C ⃗ ∣ AD = {|\vec {AB} \times \vec {AC}| \over |\vec{BC}|} A D = ∣ B C ∣ ∣ A B × A C ∣
1 2 3 4 double dis_PL (point p,point A,point B) return abs (Cross (p-A,p-B)) / len (A-B); }
直线 A B AB A B A + t v A + tv A + t v P P P Q Q Q t 0 t_0 t 0 Q P ⃗ ⊥ A B ⃗ \vec {QP} \perp \vec {AB} Q P ⊥ A B
Q = A + t 0 v ( P − Q ) ⋅ v = 0 ( P − A − t 0 v ) ⋅ v = 0 ( P − A ) ⋅ v − t 0 v ⋅ v = 0 t 0 = ( P − A ) ⋅ v v ⋅ v Q = A + t_0 v\\
(P-Q)\cdot v = 0\\
(P - A - t_0v) \cdot v = 0\\
(P-A) \cdot v - t_0v \cdot v = 0\\
t_0 = {(P-A) \cdot v\over v \cdot v}\\
Q = A + t 0 v ( P − Q ) ⋅ v = 0 ( P − A − t 0 v ) ⋅ v = 0 ( P − A ) ⋅ v − t 0 v ⋅ v = 0 t 0 = v ⋅ v ( P − A ) ⋅ v
1 2 3 4 5 point GetLineRoot (point p,point A,point B) point v = B - a; return A + v * (Dot (P-A,v) / Dot (v,v)); }
假设 直线上一点 P P P v v v Q Q Q P Q ⃗ × v \vec {PQ} \times v P Q × v Q Q Q
叉积为零,在直线上,叉积为正,Q Q Q
先找到点 P P P Q Q Q P Q PQ P Q
1 2 3 4 point Symmetry_PL (point p,point A,point B) return p + (GetLineRoot (p,A,B)-p) * 2 ; }
(这里原来有一道巨ex的题)
设两直线方程分别为: l 1 = P + t v ⃗ l_1 = P + t\vec v l 1 = P + t v l 2 = Q + t w ⃗ l_2 = Q + t\vec w l 2 = Q + t w
则: 交点在第一条直线的参数为 $ t $ ,则 t = ( P − Q ) × w ⃗ w ⃗ × v ⃗ t = {(P-Q) \times \vec w \over \vec w \times \vec v} t = w × v ( P − Q ) × w
因为是交点,所以同时满足两个直线的方程,令交点在两条直线上的参数分别为 t t t μ \mu μ
则有:P + t v ⃗ = Q + μ w ⃗ P + t \vec v = Q + \mu \vec w P + t v = Q + μ w
两边同时叉上 w ⃗ \vec w w ( P + t v ⃗ ) × w ⃗ = ( Q + μ w ⃗ ) × w ⃗ (P + t\vec v)\times \vec w = (Q + \mu\vec w)\times \vec w ( P + t v ) × w = ( Q + μ w ) × w
去括号可得: P × w ⃗ + t v ⃗ × w ⃗ = Q × w ⃗ + μ w ⃗ × w ⃗ P\times \vec w + t\vec v \times\vec w = Q\times \vec w + \mu \vec w \times \vec w P × w + t v × w = Q × w + μ w × w
又因为 w ⃗ × w ⃗ = 0 \vec w \times \vec w = 0 w × w = 0 P × w ⃗ + t v ⃗ × w ⃗ = Q × w ⃗ P \times \vec w + t\vec v \times \vec w = Q \times \vec w P × w + t v × w = Q × w
移项可得: t v ⃗ × w ⃗ = ( Q − P ) × w ⃗ t\vec v \times \vec w = (Q-P)\times \vec w t v × w = ( Q − P ) × w
所以: t = ( P − Q ) × w ⃗ w ⃗ × v ⃗ t = {(P-Q)\times \vec w\over \vec w \times \vec v} t = w × v ( P − Q ) × w
1 2 3 4 5 6 point jiao_LL (point A,point B,point C,point D) point v = B - A, w = D - C, PQ = A - C; double t = Cro (PQ,w) / Cro (w,v); return A + t * v; }
设凸 n n n P 0 P 1 . . . . P n P_0P_1....P_n P 0 P 1 . . . . P n A A A θ i = < A P i ⃗ , A P i + 1 ⃗ > \theta_i = <\vec{AP_i},\vec{AP_{i+1}}> θ i = < A P i , A P i + 1 > A A A ∑ i = 0 n − 1 θ i = 2 π \displaystyle\sum_{i=0}^{n-1} \theta_i = 2π i = 0 ∑ n − 1 θ i = 2 π
需要特判一下在多边形顶点上的情况。
1 2 3 4 5 6 7 8 9 10 11 12 point p[1005 ]; int n;bool InPloygon (point A) double alpha = 0 ; for (int i = 1 ; i <= n; i++) { if (i == n) alpha += fabs (Angle (p[i]-A,p[1 ]-A)); else alpha += fabs (Angle (p[i]-A,p[i+1 ]-A)); } return dcmp (alpha-2 * pai) == 0 ; }
例题: UVA109 SCUD Busters
给你 m m m n n n m m m
转角法和射线法?我也不知道,全家桶里的东西被偷吃了
如果用上面的转角法和射线法来判断的话,复杂度为 O ( n m ) O(nm) O ( n m )
有没有一个更高效的算法呢?我们可以用二分来做到 O ( m l o g n ) O(mlogn) O ( m l o g n )
首先任选一个点为起点,向凸多边形其他顶点做一条射线。如图:
显然,会把整个凸多边形分成 n − 2 n-2 n − 2
第二步:判断这个点是否在所有射线的包含范围之内,即判断定点是否在最左侧射线的左边或者最右侧射线的右边。
第三部:二分出定点在那个三角形内部,然后判断定点是否在这个三角形内部即可。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 int InPolygn (point p,point *a) if (Cro (p-a[1 ],a[2 ]-a[1 ]) < 0 || Cro (p-a[1 ],a[n]-a[1 ]) > 0 ) return 0 ; if (pd_PS (p,a[1 ],a[2 ]) || pd_PS (p,a[n],a[1 ])) return 2 ; int l = 2 , r = n; while (r - l > 1 ) { int mid = (l + r)>>1 ; if (Cro (p-a[1 ],a[mid]-a[1 ]) < 0 ) l = mid; else r = mid; } if (Cro (p-a[l],a[r]-a[l]) > 0 ) return 0 ; if (pd_PS (p,a[l],a[r])) return 2 ; return 1 ; }
很简单,判断点到圆心的距离和圆的半径大小即可。
我们先求出 A B AB A B A C AC A C ∠ C B A \angle CBA ∠ C B A
那么两条切线就是 直线 A B AB A B ∠ C B A \angle CBA ∠ C B A
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 int get_PC (point p,Circle c,line *v) Vector u = c.p - p; double dis = dis (p,c.p); if (dis < r) return 0 ; else if (dcmp (dis,r) == 0 ) { v[0 ] = retate (u,PI/2 ); return 1 ; } else { double ang = asin (c.r/dis); v[0 ] = retate (u,ang); v[1 ] = retate (u,-ang); return 2 ; } }
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 point get (Circle c,double a) return point (c.x+cos (a)*c.r,c.y+sin (a)*r); } double Angle (point a) return atan2 (a.x,a.y);}int getCC (Cirle c1,Cirle c2,vector<point>& sta) double d = len (c1.c-c2.c); if (cdmp (d) == 0 ) { if (dcmp (c1.r-c2.r) == 0 ) return -1 return 0 ; } if (dcmp (c1.r+c2.r-d) < 0 ) return 0 ; if (dcmp (fabs (c1.r-c2.r)-d) > 0 ) return 0 ; double alpha = Angle (c2.c-c1.c); double deta = acos ((c1.r*c1.r+d*d-c2.r*c2.r)/(2 *c1.r*d)); point p1 = get (c1,alpha-deta); point p2 = get (c1,alpha+deta); sta.push_back (p1); if (p1 == p2) return 1 ; sta.push_back (p2); return 2 ; }
三点各坐标的平均值: ( x 1 + x 2 + x 3 3 , y 1 + y 2 + y 3 3 ) ({x_1+x_2+x_3\over 3}, {y_1+y_2+y_3\over 3}) ( 3 x 1 + x 2 + x 3 , 3 y 1 + y 2 + y 3 )
因为从第一个顶点出发可以把多边形分为 n − 2 n-2 n − 2 n − 2 n-2 n − 2
因为你叉积运算的时候是带符号的,所以你计算结果可能是面积也可能是 面积的相反数,取个绝对值就行了。
在你计算复杂多边形的面积的时候,多算的面积就会被叉积的符号抵消掉。
1 2 3 4 5 6 double PloyArea (point *a,int n) double res = 0 ; for (int i = 2 ; i < n; i++) res += Cro (a[i]-a[1 ],a[i+1 ]-a[1 ]); return abs (res / 2 ); }
P1183 多边形的面积
用来求定积分。
当函数极为鬼畜的时候,我们采用二次拟合的形式,当精度相等的时候,便视为相等。
假如说我们要求 ∫ a b f ( x ) d ( x ) \int_{a}^{b} f(x) d(x) ∫ a b f ( x ) d ( x )
我们可以把这个函数近似成矩形和梯形和等等。我们为了保证准确性,这里选择二次函数来拟合曲线。
设 f ( x ) ≈ A x 2 + B x + C f(x) \approx Ax^2 + Bx+ C f ( x ) ≈ A x 2 + B x + C
∫ a b f ( x ) d ( x ) ≈ ∫ a b A x 2 + B x + C \int_{a}^{b} f(x)d(x) \approx \int_{a}^{b} Ax^2+Bx+C ∫ a b f ( x ) d ( x ) ≈ ∫ a b A x 2 + B x + C
= ∫ a b A x 2 + ∫ a b B x + ∫ a b C = \int_{a}^{b} Ax^2 + \int_{a}^{b} Bx + \int_{a}^{b} C = ∫ a b A x 2 + ∫ a b B x + ∫ a b C
= A 3 x 3 + B 2 x 2 + C x ∣ a b ={A\over 3}x^3 + {B\over 2}x^2 + Cx|_{a}^{b} = 3 A x 3 + 2 B x 2 + C x ∣ a b
= ( a − b ) 6 ( 2 A ( a 2 + a b + b 2 ) + 3 B ( a + b ) + 6 C ) ={(a-b)\over 6} (2A(a^2+ab+b^2) + 3B(a+b) + 6C) = 6 ( a − b ) ( 2 A ( a 2 + a b + b 2 ) + 3 B ( a + b ) + 6 C )
= a − b 6 ( ( A a 2 + B a + c ) + ( A b 2 + B b + c ) + 4 ( ( a + b 2 ) 2 + a + b 2 + c ) ) ={a-b\over 6} ((Aa^2+Ba+c) + (Ab^2+Bb+c) + 4(({a+b\over 2})^2 + {a+b\over 2} + c)) = 6 a − b ( ( A a 2 + B a + c ) + ( A b 2 + B b + c ) + 4 ( ( 2 a + b ) 2 + 2 a + b + c ) )
$ = {a-b\over 6} (f(a) + f(b) + 4f({a+b\over 2}))$
然后就有个结论: $$\int_abf(x)dx\approx\int_a {b}(Ax^2+Bx+C)dx=\frac{(a-b)(f(a)+f(b)+4f(\frac{a+b}{2}))}{6}$$
这样直接算的话,我们的精度误差会很大,我们考虑吧 [ a , b ] [a,b] [ a , b ] [ a , m i d ] , [ m i d , b ] [a,mid],[mid,b] [ a , m i d ] , [ m i d , b ]
当 ∫ a m i d f ( x ) d ( x ) + f m i d b f ( x ) d ( x ) = f a b f ( x ) d ( x ) + e p s \int_{a}^{mid} f(x)d(x) + f_{mid}^{b}f(x)d(x) = f_{a}^{b}f(x)d(x) + eps ∫ a m i d f ( x ) d ( x ) + f m i d b f ( x ) d ( x ) = f a b f ( x ) d ( x ) + e p s
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 double f (double x) return 1.0 *(c*x+d)/(a*x+b);}double calc (double l,double r) double mid = (l +r)/2.0 ; return (r-l)*(f (l)+f (r)+4 *f (mid))/6 ; } double simpon (double l,double r,double last) double mid = (l + r)/2 ; double lx = calc (l,mid), rx = calc (mid,r); if (fabs (lx + rx - last) < eps) return lx+rx; else return simpon (l,mid,lx) + simpon (mid,r,rx); } int main () scanf ("%lf%lf%lf%lf%lf%lf" ,&a,&b,&c,&d,&l,&r); printf ("%.6lf\n" ,simpon (l,r,calc (l,r))); return 0 ; }
例题: 板子1 板子2
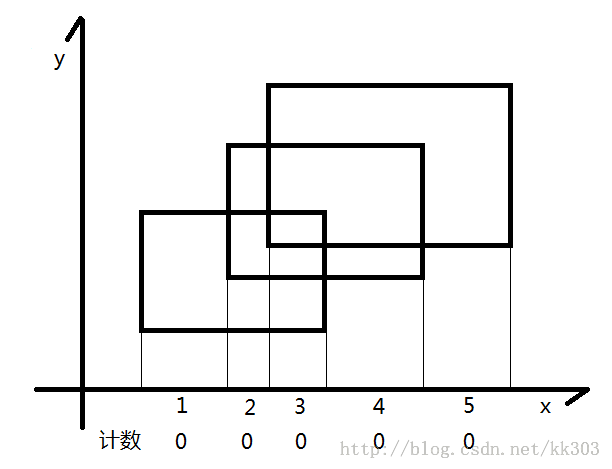
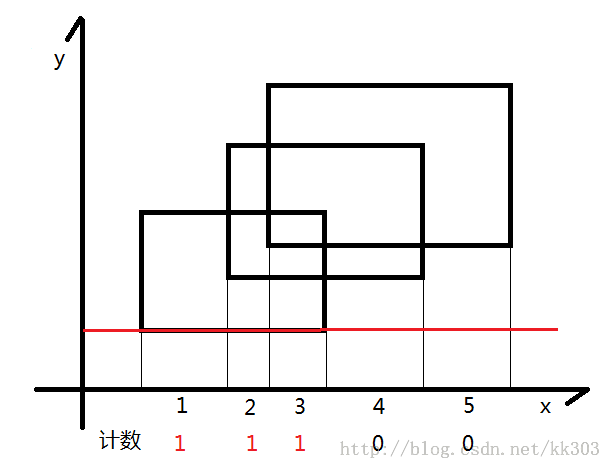
其实这个算法挺冷门的,学了这个关键是做 这道题 (柠檬树上懵逼果,柠檬树下你和我 )
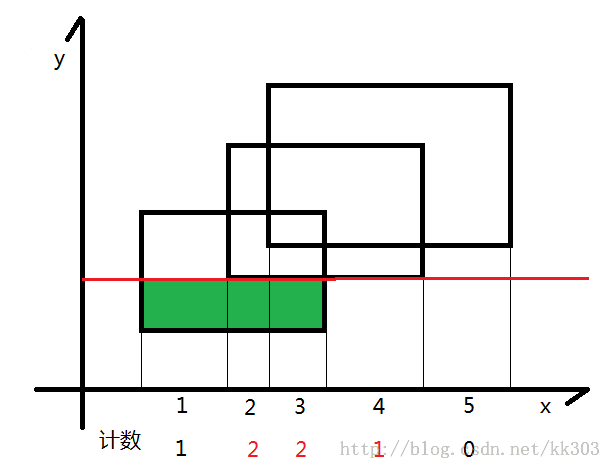
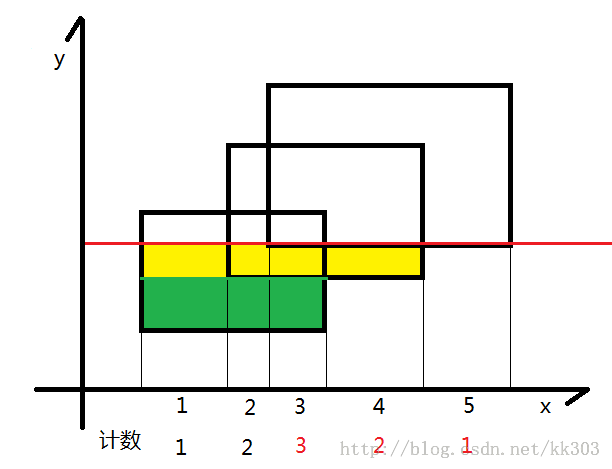
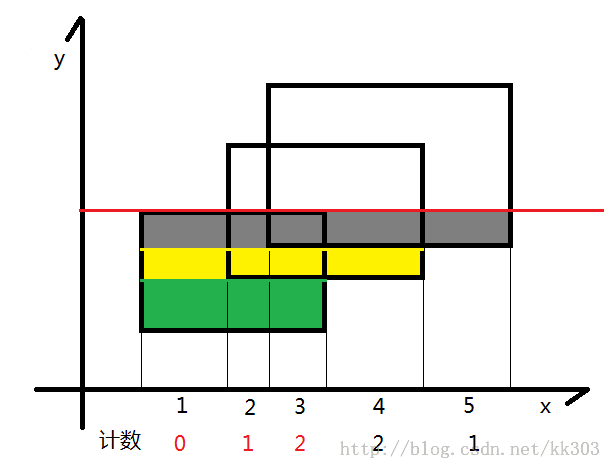
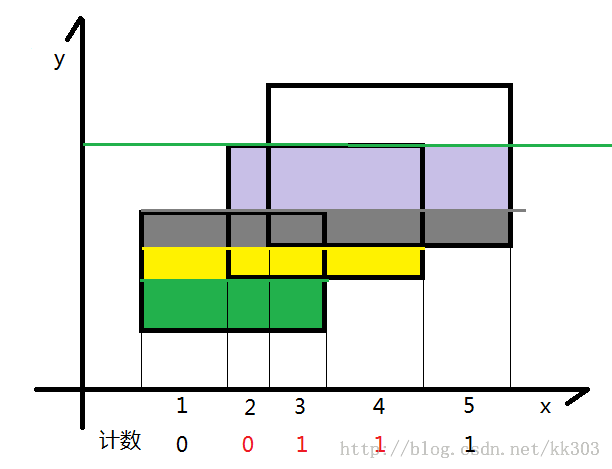
全家桶里也没有,我稍微看了看,大概就是一条扫描线进行扫描,然后
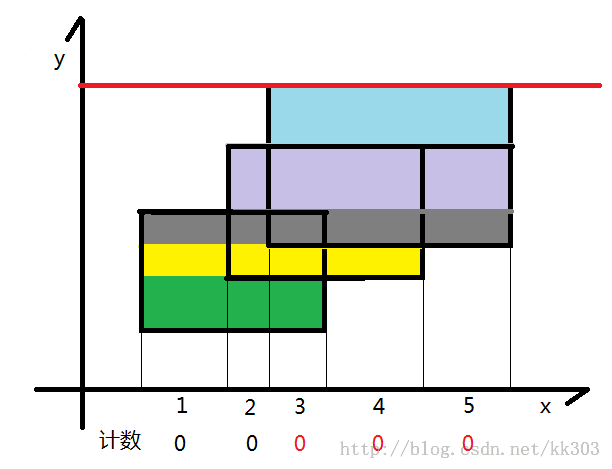
离散化: 这些技巧都是老生常谈的了, 不然浮点数怎么建树, 离散化坐标就可以了
扫描线: 首先把矩形按轴分成两条边, 上边和下边, 对轴建树, 扫描线可以看成一根平行于轴的直线.
线段树: 用于动态维护扫描线在往上走时, 轴哪些区域是有合法面积的
这种线段树是不用的, 因为不用, 为啥不用, 因为没有查询操作
不太懂www
不如我们再看看图?
没咋看的代码(不如先放放吧):
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 using namespace std;const int N = 205 , INF = 0x3f3f3f3f , MOD = 1e9 + 7 ; int n;struct Seg { double l, r, h; int d; Seg () {} Seg (double l, double r, double h, int d): l (l), r (r), h (h), d (d) {} bool operator < (const Seg& rhs) const {return h < rhs.h;} } a[N]; int cnt[N << 2 ]; double sum[N << 2 ], all[N]; #define lson l, m, rt << 1 #define rson m + 1, r, rt << 1 | 1 void push_up (int l, int r, int rt) if (cnt[rt]) sum[rt] = all[r + 1 ] - all[l]; else if (l == r) sum[rt] = 0 ; else sum[rt] = sum[rt << 1 ] + sum[rt << 1 | 1 ]; } void update (int L, int R, int v, int l, int r, int rt) if (L <= l && r <= R) { cnt[rt] += v; push_up (l, r, rt); return ; } int m = l + r >> 1 ; if (L <= m) update (L, R, v, lson); if (R > m) update (L, R, v, rson); push_up (l, r, rt); } int main () int kase = 0 ; while (scanf ("%d" , &n) == 1 && n) { for (int i = 1 ; i <= n; ++i) { double x1, y1, x2, y2; scanf ("%lf%lf%lf%lf" , &x1, &y1, &x2, &y2); a[i] = Seg (x1, x2, y1, 1 ); a[i + n] = Seg (x1, x2, y2, -1 ); all[i] = x1; all[i + n] = x2; } n <<= 1 ; sort (a + 1 , a + 1 + n); sort (all + 1 , all + 1 + n); int m = unique (all + 1 , all + 1 + n) - all - 1 ; memset (cnt, 0 , sizeof cnt); memset (sum, 0 , sizeof sum); double ans = 0 ; for (int i = 1 ; i < n; ++i) { int l = lower_bound (all + 1 , all + 1 + m, a[i].l) - all; int r = lower_bound (all + 1 , all + 1 + m, a[i].r) - all; if (l < r) update (l, r - 1 , a[i].d, 1 , m, 1 ); ans += sum[1 ] * (a[i + 1 ].h - a[i].h); } printf ("Test case #%d\nTotal explored area: %.2f\n\n" , ++kase, ans); } return 0 ; }
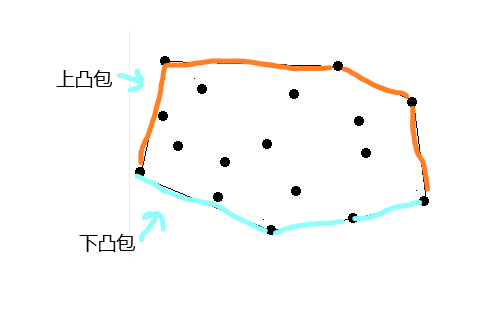
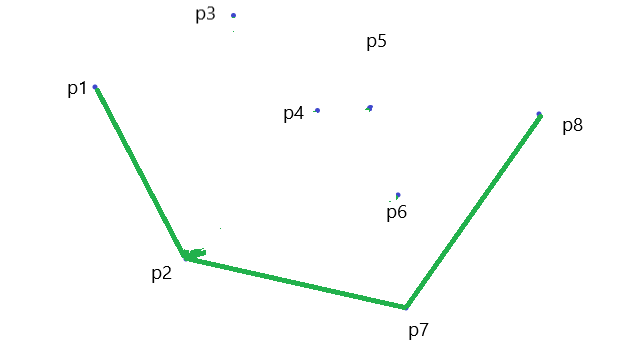
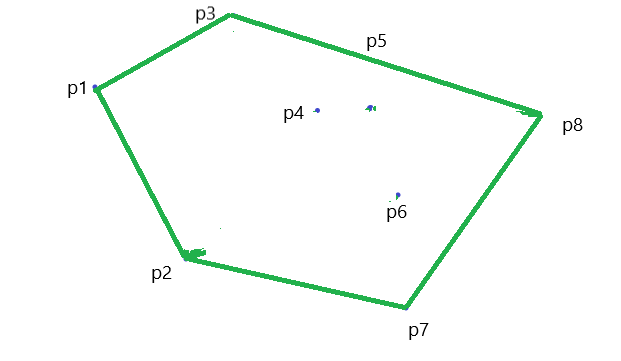
现在平面上有一堆点,让你找到一个点集,依次连接这些点,组成一个多边形,且所有的点都在这个多边形内部或边上,这个多边形我们称之为凸包。
就相当于平面上有很多钉子,你拿一个橡皮筋一围,围出来的多边形就是凸包。
大概张这个样子:
然后观察一下这个凸包,你会发现一下很好玩的性质。
上凸壳中的点的斜率是单调递减的。
下凸壳中的点的斜率的单点递增的。
这个性质有什么用呢? 他主要是用来斜率优化的。
首先我们发现最下面的一个点肯定会在凸包上。所以一开始我们可以通过一遍扫描把这一个点找出来记做点 A A A
第二步:把所有的点按 A p i Ap_i A p i A A A
第三步:维护一个栈,来保存当前凸包的形态。将每个点按顺序依次加入凸包中。如果当前这个点 P P P B , C B,C B , C B C ⃗ \vec{BC} B C P C ⃗ \vec{PC} P C B B B
顺时针的情况为什么不考虑呢?因为可能是在拐弯处。
口胡说的不太清楚,拿一些图演示一下。
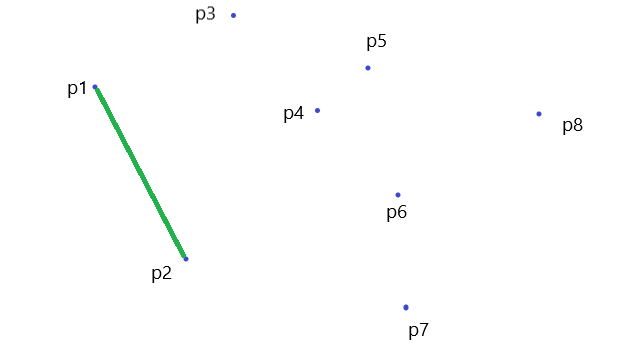
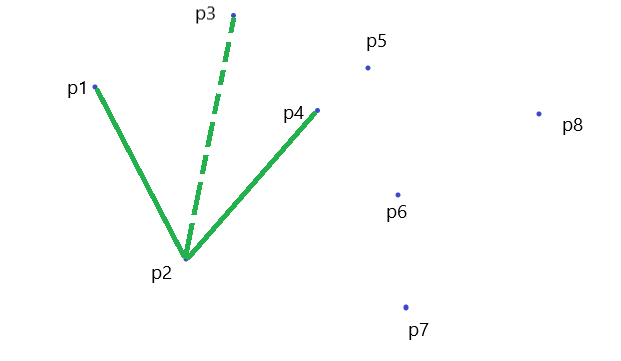
(1)首先给你一堆点, 最下面的点 p 1 p_1 p 1
(2)然后 P 2 P_2 P 2
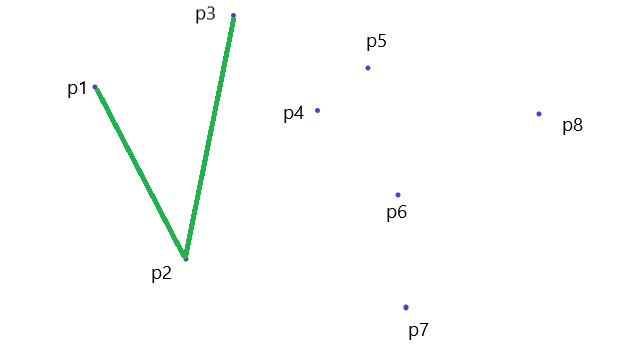
(3)之后因为 P 2 P 1 ⃗ \vec {P_2P_1} P 2 P 1 P 3 P 1 ⃗ \vec{P_3P_1} P 3 P 1
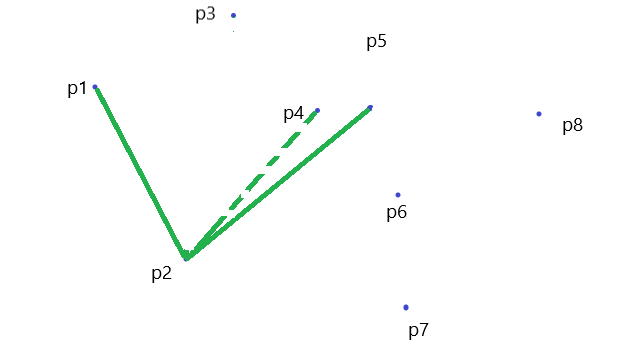
(4)之后 P 3 P 2 ⃗ \vec {P_3P_2} P 3 P 2 P 4 P 2 ⃗ \vec{P_4P_2} P 4 P 2 P 3 P_3 P 3 P 2 P_2 P 2 P 4 P_4 P 4
(5) P 5 P_5 P 5
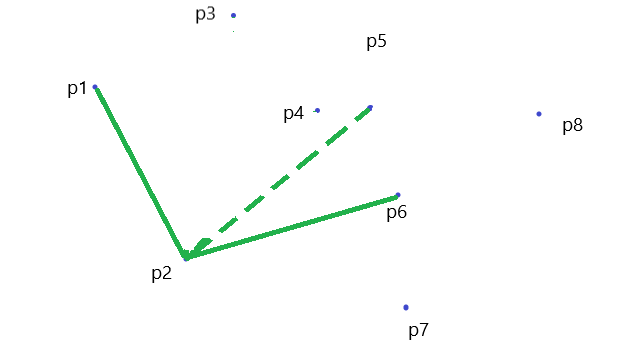
(6) P 6 P_6 P 6 P 5 P 4 ⃗ \vec{P_5P_4} P 5 P 4 P 6 P 4 ⃗ \vec{P_6P_4} P 6 P 4 P 5 P_5 P 5
(7) 又发现 P 4 P 2 ⃗ \vec{P_4P_2} P 4 P 2 P 4 P_4 P 4
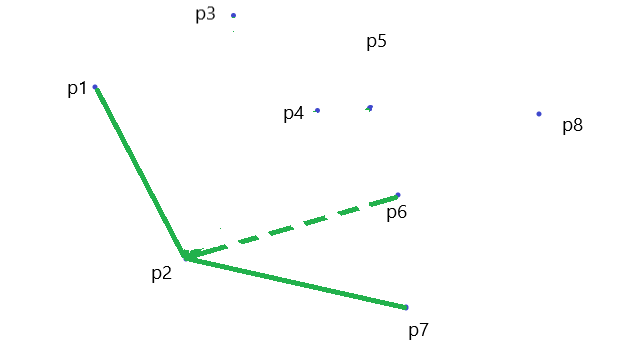
(8) P 7 P_7 P 7
(9)P 8 P_8 P 8
(10) 最后就连到了 起点 P 1 P_1 P 1
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;const double eps = 1e-8 ;int n,top;double ans;struct point { double x,y; point (double X = 0 , double Y = 0 ){x = X, y = Y;} }p[100010 ],sta[100010 ]; point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double b){return point (a.x*b,a.y*b);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}int dcmp (double x) if (fabs (x) < eps) return 0 ; if (x > 0 ) return 1 ; return -1 ; } double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x) + (a.y-b.y) * (a.y-b.y)); } bool comp (point a,point b) double m = Cro (a-p[1 ],b-p[1 ]); if (dcmp (m) > 0 ) return 1 ; if (dcmp (m) == 0 && dcmp (dis (a,p[1 ])-dis (b,p[1 ])) < 0 ) return 1 ; return 0 ; } int main () scanf ("%d" ,&n); for (int i = 1 ; i <= n; i++) { scanf ("%lf%lf" ,&p[i].x,&p[i].y); if (dcmp (p[i].y-p[1 ].y) < 0 ) swap (p[1 ],p[i]); else if (dcmp (p[i].y-p[1 ].y) == 0 && dcmp (p[i].x-p[1 ].x) < 0 ) swap (p[1 ],p[i]); } sort (p+2 ,p+n+1 ,comp); sta[++top] = p[1 ]; sta[++top] = p[2 ]; for (int i = 3 ; i <= n; i++) { while (top >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) <= 0 ) top--; sta[++top] = p[i]; } for (int i = 1 ; i < top; i++) ans += dis (sta[i],sta[i+1 ]); ans += dis (sta[top],sta[1 ]); printf ("%.2lf\n" ,ans); return 0 ; }
例题: 模板 信用卡凸包
这个其实是上面那种算法的变种。一般来说这个是要比上面那个算法是快的,并且精度也比较高。
第一步:先把所有的点按横坐标为第一关键字纵坐标为第二关键字排序(这个和上面是不同的,这也是水平序比极角序要快的原因)。
第二步:从前往后扫一遍得到下凸壳。
第二步:从后往前倒着扫一遍得到上凸壳。
来张图模拟一下:
排完序之后的点集如图:
首先,我们先把前两个点入栈
因为 p 1 p_1 p 1
P 2 P_2 P 2
$ P_1P_2P_3$ 组成的是一个凸多边形,所以 P 3 P_3 P 3
P 4 P_4 P 4 P 3 P_3 P 3 P 2 P 3 P_2P_3 P 2 P 3 P 3 P_3 P 3 P 2 P_2 P 2 P 4 P_4 P 4
同理: P 4 P_4 P 4 P 5 P_5 P 5
P 5 P_5 P 5 P 6 P_6 P 6
P 6 P_6 P 6 P 7 P_7 P 7
P 8 P_8 P 8
有木有发现一个很严重的问题,扫描到最后,我们只求出来个下凸壳。
那么上凸壳怎么求呢,很简单,倒着再跑一遍即可。
最后求出来的凸包长这样:
最后栈中的元素为: P 1 , P 2 , P 7 , P 8 , P 8 , P 5 , P 3 , P 1 P_1,P_2,P_7,P_8,P_8,P_5,P_3,P_1 P 1 , P 2 , P 7 , P 8 , P 8 , P 5 , P 3 , P 1
你会发现起点和终点在栈中出现了两次,但自己到自己的距离为零,所以不会对答案产生影响。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 #include <iostream> #include <cstdio> #include <cmath> #include <algorithm> using namespace std;const int N = 1e5 +10 ;const double eps = 1e-8 ;int n,top;double ans;struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }p[N],sta[N]; point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)); } int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } bool comp (point a,point b) if (dcmp (a.x-b.x) == 0 ) return a.y < b.y; return a.x < b.x; } int main () scanf ("%d" ,&n); for (int i = 1 ; i <= n; i++) scanf ("%lf%lf" ,&p[i].x,&p[i].y); sort (p+1 ,p+n+1 ,comp); sta[++top] = p[1 ]; sta[++top] = p[2 ]; for (int i = 3 ; i <= n; i++) { while (top >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) < 0 ) top--; sta[++top] = p[i]; } int last = top; sta[++top] = p[n]; sta[++top] = p[n-1 ]; for (int i = n-2 ; i >= 1 ; i--) { while (top - last >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) < 0 ) top--; sta[++top] = p[i]; } for (int i = 1 ; i < top; i++) ans += dis (sta[i],sta[i+1 ]); printf ("%.2lf\n" ,ans); return 0 ; }
先来道板子题: 防线修建
我们考虑加入一个点之后,会对当前的凸包产生什么影响。
如图我们当前凸包长这样:
当我们加入一个点 G G G
根据静态凸包的构造方法(Anderw扫描法):
将各点坐标以横坐标为第一关键字,纵坐标为第二关键字排序。
维护一下上凸壳和下凸壳。拿维护上凸壳举例,注意到如果之前有两点 A A A B B B C C C A B → × A C → ≥ 0 \overrightarrow{AB} \times \overrightarrow{AC} ≥0 A B × A C ≥ 0 B B B A C AC A C B B B
我们拿 s e t set s e t
显然每加入一个点,被删除的只能是他在 s e t set s e t
这个题我们离线一下,倒着做,就只需要动态维护一个上凸壳,比较好写点.
感觉自己写的时候,尽量画个图参照着写,不然的话你就很容易搞混。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> #include <set> using namespace std;const int N = 1e5 +10 ;const double eps = 1e-8 ;int n,m,cntq;double x,y,last;bool vis[N];struct node { int opt,c; double ans; }q[N]; struct point { double x,y; double ang; point (){} point (double a,double b){x = a, y = b;} friend bool operator < (point a,point b) { if (a.x == b.x) return a.y < b.y; return a.x < b.x; } }p[N],o; int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}set<point> s; void insert (point now) set<point>::iterator itl,itr,tmp; itr = s.lower_bound (now); itl = itr; --itl; if (Cro (now-*itl,*itr-*itl) > 0 ) return ; last -= dis (*itl,*itr); while (itr != s.end ()) { tmp = itr; ++itr; if (itr == s.end () || Cro (now-*itr,*tmp-*itr) < 0 ) break ; last -= dis (*tmp,*itr); s.erase (tmp); } while (itl != s.begin ()) { tmp = itl; --itl; if (Cro (now-*itl,*tmp-*itl) > 0 ) break ; last -= dis (*tmp,*itl); s.erase (tmp); } s.insert (now); itl = itr = s.find (now); --itl; ++itr; last += dis (now,*itl) + dis (now,*itr); } int main () scanf ("%d%lf%lf" ,&n,&x,&y); point a = point (0 ,0 ); point b = point (n,0 ); point c = point (x,y); s.insert (a); s.insert (b); s.insert (c); last += dis (b,c) + dis (c,a); scanf ("%d" ,&m); for (int i = 1 ; i <= m; i++) scanf ("%lf%lf" ,&p[i].x,&p[i].y); scanf ("%d" ,&cntq); for (int i = 1 ; i <= cntq; i++) { scanf ("%d" ,&q[i].opt); if (q[i].opt == 1 ) { scanf ("%d" ,&q[i].c); vis[q[i].c] = 1 ; } } for (int i = 1 ; i <= m; i++) if (!vis[i]) insert (p[i]); for (int i = cntq; i >= 1 ; i--) { if (q[i].opt == 1 ) insert (p[q[i].c]); else q[i].ans = last; } for (int i = 1 ; i <= cntq; i++) if (q[i].opt == 2 ) printf ("%.2lf\n" ,q[i].ans); return 0 ; }
这个他的原理是什么呢?主要是在一个凸包上,他的很多函数都是单峰的。比如固定一条多边形的边,其他的点和这条边组成的面积是单峰的。
凸包的直径:定义凸包上任意两点之间距离的最大值。
怎么求? 暴力枚举 $O(n^2) $
有一个结论:如果一个点到一条边的距离最远,那么点到线段两端点中一个的距离一定是最远的。
优化一下,首先我们可以固定一条边,然后点到这条边的距离是一个单峰的函数,显然我们是可以三分出来的,复杂度 O ( n l o g n ) O(nlogn) O ( n l o g n )
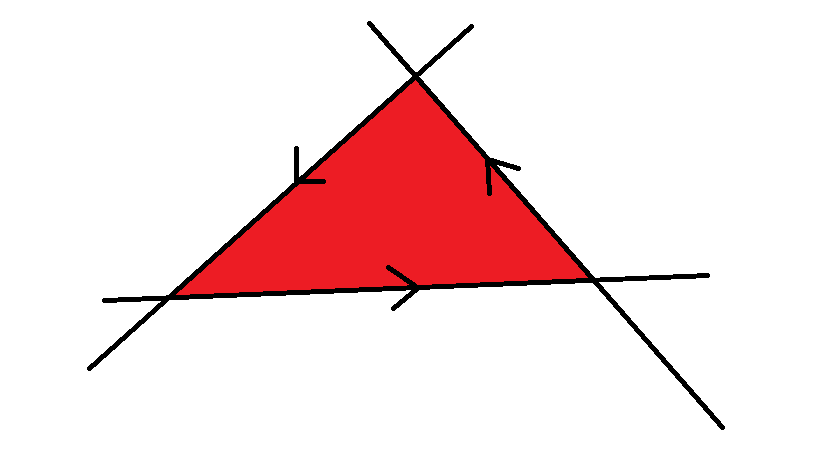
继续优化,旋转卡壳利用了一个更好的性质,假设说我们固定的这条边是不断滚动的,如图:
先固定 B C BC B C C D CD C D D E DE D E
你会发现,当你固定 B C BC B C E E E F F F C D CD C D E E E F F F
也就是说:我们的边是顺时针的枚举,而此时距离他们最远的点也是顺时针的出现!!
然后我们就可以通过双指针扫一遍就可以得到这个凸包的直径。
基本上旋转卡壳就是这么一个流程。
一个动图演示一下:
1 2 3 4 5 6 7 8 9 10 11 double Get_zhijing () double res = 0 ; sta[top + 1 ] = p[1 ]; for (int i = 1 , j = 2 ; i <= top; i++) { while (Cro (sta[i]-sta[j],sta[i+1 ]-sta[j]) < Cro (sta[i]-sta[j+1 ],sta[i+1 ]-sta[j+1 ])) j = j % top + 1 ; res = max (res,max (dis (sta[i],sta[j]),dis (sta[i+1 ],sta[j]))); } return res; }
例题:旋转卡壳
让你找一个矩形使得这个矩形能覆盖住点集中所有的点,且矩形的,面积最小。
怎么求?暴力枚举 O ( n 4 ) O(n^4) O ( n 4 )
考虑到这个矩形他肯定有一条边是会和凸包的一条边重合的(这个并不好证,但反正他是正确的)
当我们固定一条边的时候,我们还需要知道,每个点到这条边投影的最大值和最小值(矩形的左右侧),和点到这条边距离的最大值(矩形的上底边)。
结合图理解一下:
假如说,我们要求这个多边形的最小矩形覆盖:
当我们固定 D E DE D E
很容易发现,矩形的高就是点到 E D ED E D E D ED E D
这三个东西,都可以拿旋转卡壳维护出来 (也可以在凸包上三分),复杂度 O ( n ) O(n) O ( n )
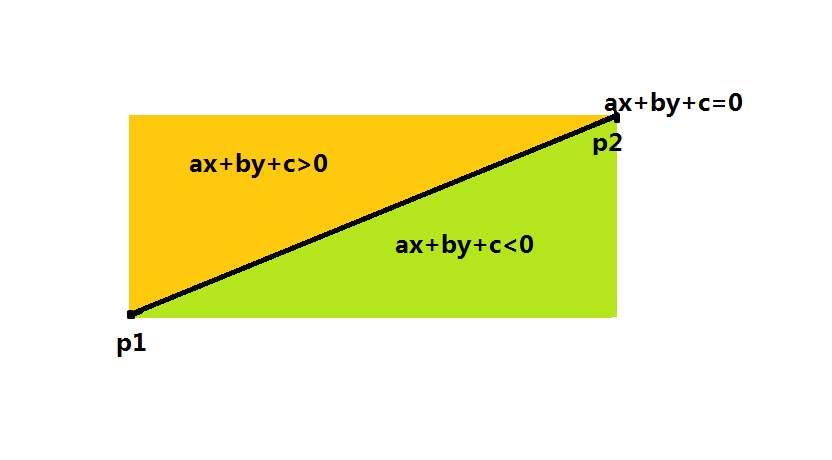
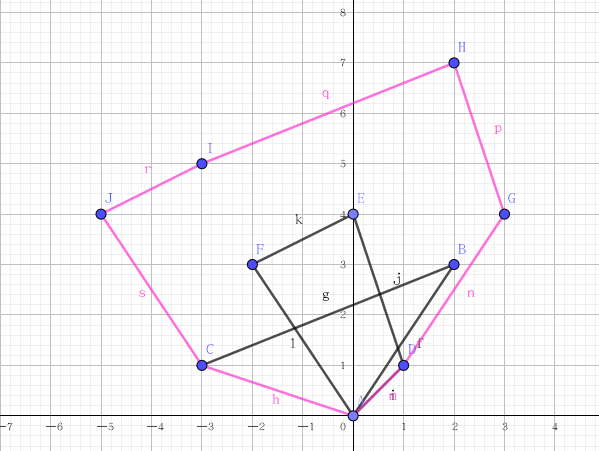
如图:一条直线把平面分为了两部分,我们通常把直线的左侧平面称为他的半平面。
如下图所示,直线 P 1 P 2 P_1P_2 P 1 P 2
注意直线的方向不同,所构成的半平面也是不同的,因此在做半平面交的时候要注意一下方向问题。
半平面交:多个半平面同时覆盖的区域叫做半平面交,如图,红色区域就是那三条直线的半平面交。
我们还可以观察到,第一个图中直线 P 1 P 2 P_1P_2 P 1 P 2 a x + b y + c > 0 ax+by+c > 0 a x + b y + c > 0 P 2 P 1 P_2P_1 P 2 P 1
则是 a x + b y + c < 0 ax+by+c < 0 a x + b y + c < 0
所以半平面交的本质就是方程组:$a_ix+b_iy+c > 0 $ 或 a i x + b i y + c < 0 a_ix+b_iy+c < 0 a i x + b i y + c < 0
一般半平面交的题都是让你列出等式,然后构造半平面交求解。
怎么求?
我们发现半平面交的区域是一个凸多边形(或者空集,线段)。
计算半平面交的一个好方法就是增量法,即初始答案为整个平面,然后注意加入各个平面,维护当前的半平面交。
一开始我们像凸包那样把所有的直线按极角排一下序,这样可以保证我们当前合法的直线的交就是我们的半平面交,然后考虑依次加入每一条线段。
我们开两个队列,一个维护合法的直线,另一个维护半平面交的交点,以便确定半平面交的形态。
如果前面两条合法直线 A , B A,B A , B C C C A A A B B B C C C B B B A A A B B B C C C A C AC A C
如图:
如果交点 G G G C C C B B B
因为最后我们要围成一个多边形,所以你现在加入的这一条直线可能会使对首的直线不合法,举个例子:
当我们加入 D H DH D H A B AB A B
那么我们需要开个双端队列来维护一下,每次加入一条直线的时候,检查队头和队尾,去除不合法的元素即可。
当我们半平面交是无限大的时候怎么办?如图:
这时候我们在一开始可以人为地加入四条边界直线,这样半平面交就不会无限大啦。
愉快的代码时间:
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;const double lim = 1000.0 ;const double eps = 1e-8 ;const int N = 1e5 +10 ;int n,m,l,r,cnt;double area;struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }sta[N],p[N],ans[N]; typedef point Vector;struct line { point x,y; double ang; line (){} line (point a,point b) { x = a; y = b; ang = atan2 (b.y-a.y,b.x-a.x); } }L[N],q[N]; point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}bool OnRight (point p,line s) return Cro (p-s.x,s.y-s.x) > 0 ;}int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } bool comp (line a,line b) if (a.ang == b.ang) return OnRight (b.x,a); return a.ang < b.ang; } point Root_LL (line a,line b) Vector v = a.y-a.x, u = b.y-b.x, w = a.x-b.x; double t = Cro (w,u)/Cro (u,v); return a.x + v * t; } int HPI () int m = 0 ; sort (L+1 ,L+cnt+1 ,comp); l = 1 , r = 1 ; q[1 ] = L[1 ]; for (int i = 2 ; i <= cnt; i++) { if (dcmp (L[i].ang - L[i-1 ].ang) == 0 ) continue ; while (l < r && OnRight (sta[r-1 ],L[i])) r--; while (l < r && OnRight (sta[l],L[i])) l++; q[++r] = L[i]; if (l < r) sta[r-1 ] = Root_LL (q[r],q[r-1 ]); } while (l < r && OnRight (sta[r-1 ],q[l])) r--; while (l < r && OnRight (sta[l],q[r])) l++; if (r - l + 1 <= 2 ) return 0 ; sta[r] = Root_LL (q[l],q[r]); for (int i = l; i <= r; i++) ans[++m] = sta[i]; return m; } int main () scanf ("%d" ,&n); for (int i = 1 ; i <= n; i++) { scanf ("%d" ,&m); for (int j = 1 ; j <= m; j++) scanf ("%lf%lf" ,&p[j].x,&p[j].y); for (int j = 1 ; j < m; j++) L[++cnt] = line (p[j],p[j+1 ]); L[++cnt] = line (p[m],p[1 ]); } point a = point (-lim,lim); point b = point (-lim,-lim); point c = point (lim,-lim); point d = point (lim,lim); L[++cnt] = line (a,b); L[++cnt] = line (b,c); L[++cnt] = line (c,d); L[++cnt] = line (d,a); n = HPI (); for (int i = 2 ; i < n; i++) area += Cro (ans[i]-ans[1 ],ans[i+1 ]-ans[1 ]); printf ("%.3lf\n" ,area/2 ); return 0 ; }
随机增量算法是计算几何的一个重要算法,它对理论知识要求不高,算法时间复杂度低,应用范围广大。(扯淡 )
增量法 (Incremental Algorithm) 的思想与第一数学归纳法类似,它的本质是将一个问题化为规模刚好小一层的子问题。解决子问题后加入当前的对象。写成递归式是: T ( n ) = T ( n − 1 ) + g ( n ) T(n) = T(n-1)+g(n) T ( n ) = T ( n − 1 ) + g ( n )
直接看题吧:最小圆覆盖
怎么说呢?不会做,直接看题解。
首先,假设说我们已经求出来了前 i − 1 i-1 i − 1 i i i i i i i i i
这时候我们需要求出过点 i i i i − 1 i-1 i − 1
由于三点确定一个圆,所以我们还需要确定一下其余的两个点。
第一步:不断枚举不在最小圆上的点 j j j i + j 2 i+j\over 2 2 i + j d i s ( i , j ) dis(i,j) d i s ( i , j ) i , j i,j i , j
第三步:枚举剩下的 j − 1 j-1 j − 1 k k k k k k i , j , k i,j,k i , j , k i i i k k k
复杂度分析:
上述的是期望复杂度,一般情况下出题人可能会造一些数据卡掉这种解法。
肿么办,random_shuffle 一下即可。 n 3 n^3 n 3
至于怎么求过三个点的最小圆,你可以尝试解方程(反正太复杂了,我没看懂,第二篇题解有证明),你也可以做中垂线,求两条直线的交点。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 #include <bits/stdc++.h> using namespace std;const double eps = 1e-15 ;int n;double r;struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }p[100010 ],o; typedef point Vector;struct line { point x,y; line (){} line (point a,point b){x = a, y = b;} }; point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}point retate (point a) {return point (a.y,-a.x);}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)); } int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } line ZhongChuiXian (point a,point b) point v = retate (b-a); point A = point ((a.x+b.x)/2 ,(a.y+b.y)/2 ); return line (A,v); } point Root_LL (line a,line b) Vector v = a.y, u = b.y, w = a.x-b.x; double t = Cro (w,u)/Cro (u,v); return a.x + v * t; } point get (point a,point b,point c) line s1 = ZhongChuiXian (a,b); line s2 = ZhongChuiXian (b,c); return Root_LL (s1,s2); } int main () srand (time (0 )); rand (); scanf ("%d" ,&n); for (int i = 1 ; i <= n; i++) scanf ("%lf%lf" ,&p[i].x,&p[i].y); for (int i = 1 ; i <= n; i++) { int l = rand () % n + 1 ; int r = rand () % n + 1 ; swap (p[l],p[r]); } o = p[1 ]; r = 0 ; for (int i = 2 ; i <= n; i++) { if (dcmp (dis (p[i],o)-r) <= 0 ) continue ; o = p[i]; r = 0 ; for (int j = 1 ; j < i; j++) { if (dcmp (dis (p[j],o)-r) <= 0 ) continue ; o.x = (p[i].x+p[j].x)/2 ; o.y = (p[i].y+p[j].y)/2 ; r = dis (p[i],o); for (int k = 1 ; k < j; k++) { if (dcmp (dis (p[k],o)-r) <= 0 ) continue ; o = get (p[i],p[j],p[k]); r = dis (p[i],o); } } } printf ("%.10lf\n" ,r); printf ("%.10lf %.10lf\n" ,o.x,o.y); return 0 ; }
例题:信号塔
太难了,弃坑。有兴趣的可以到 wiki 上了解一下。
就是说,平面上有两个凸包 A A A B B B
每个凸包可以看做一个点集。
定义两个凸包的闵可夫斯基和 $A+B = {\alpha + \beta }, \alpha\in A,\beta\in B $ 。
也就是说我们从 A A A B B B
如下图,粉色区域就是三角形和四边形的闵可夫斯基和。
结论1:闵可夫斯基和是凸性的。
证明:
一个多边形是凸性的,等价于 α , β ∈ A , x β + ( 1 − x ) α ∈ A \alpha ,\beta\in A, x\beta+(1-x)\alpha \in A α , β ∈ A , x β + ( 1 − x ) α ∈ A
也就是说如果 α \alpha α β \beta β α β \alpha\beta α β
有 α 1 α 2 ∈ A , β 1 β 2 ∈ B \alpha_1\alpha_2\in A,\beta_1\beta_2\in B α 1 α 2 ∈ A , β 1 β 2 ∈ B α 1 + β 1 , α 2 + β 2 ∈ A + B \alpha_1+\beta_1,\alpha_2+\beta_2 \in {A+B} α 1 + β 1 , α 2 + β 2 ∈ A + B
那么则有 $(\alpha_1+\beta_1)x+(1-x)\ (\alpha_2+\beta_2) = \alpha_1x+(1-x)\alpha_2 + \beta_1x+(1-x)\beta_2 $
其中 α 1 x + ( 1 − x ) α 2 ∈ A \alpha_1x+(1-x)\alpha_2\in A α 1 x + ( 1 − x ) α 2 ∈ A β 1 x + ( 1 − x ) β 2 ∈ B \beta_1x+(1-x)\beta_2\in B β 1 x + ( 1 − x ) β 2 ∈ B ( α 1 + β 1 ) x + ( 1 − x ) ( α 2 + β 2 ) ∈ A + B (\alpha_1+\beta_1)x+(1-x)\ (\alpha_2+\beta_2)\in A+B ( α 1 + β 1 ) x + ( 1 − x ) ( α 2 + β 2 ) ∈ A + B
结论2:任意一条凸包 A A A B B B
证明:
一条线段在一个凸包上,等价于凸包上有一条线段和这一条线段的斜率相等,且线段外侧没有其他的点。
还是那刚才的图来举例:
比如说我们确定 α 1 α 2 \alpha_1\alpha_2 α 1 α 2 A + B A+B A + B B B B α 1 α 2 \alpha_1 \alpha_2 α 1 α 2 α 1 + β \alpha_1+\beta α 1 + β α 2 + β \alpha_2+\beta α 2 + β A + B A+B A + B α 1 α 2 \alpha_1\alpha_2 α 1 α 2 α 1 α 2 \alpha_1\alpha_2 α 1 α 2 β \beta β B B B
闵可夫斯基和的求法:
假设我们有 n n n A A A m m m B B B A A A B B B A i A i + 1 ⃗ \vec{A_iA_{i+1}} A i A i + 1 B i B i + 1 ⃗ \vec{B_iB_{i+1}} B i B i + 1 2 2 2 A A A B B B
首先,我们把每条边按斜率大小 O ( n ) O(n) O ( n ) A A A B B B
1 2 3 4 5 6 7 8 9 10 void Minkowski () for (ll i=1 ;i<n;i++) s1[i]=C1[i+1 ]-C1[i];s1[n]=C1[1 ]-C1[n]; for (ll i=1 ;i<m;i++) s2[i]=C2[i+1 ]-C2[i];s2[m]=C2[1 ]-C2[m]; A[tot=1 ]=C1[1 ]+C2[1 ]; ll p1=1 ,p2=1 ; while (p1<=n&&p2<=m) ++tot,A[tot]=A[tot-1 ]+(s1[p1]*s2[p2]>=0 ?s1[p1++]:s2[p2++]); while (p1<=n) ++tot,A[tot]=A[tot-1 ]+s1[p1++]; while (p2<=m) ++tot,A[tot]=A[tot-1 ]+s2[p2++]; }
例题: JSOI2018战争
这个题让我们判断 A + α A+\alpha A + α B B B
对于 A A A x x x B B B y y y α = y − x \alpha = y-x α = y − x
则不合法的 $\alpha $ 的集合就是 C = { y − x } , y ∈ B , x ∈ A C= \{y-x\},y\in B,x\in A C = { y − x } , y ∈ B , x ∈ A
我们把 A A A B B B A A A
每次询问判断一个点是否在这个凸包里面,二分即可。
这个当公式记就好了,一般来说没什么用。
给定顶点均为整点的简单多边形,皮克指出了其面积 A A A i i i b b b A = i + b 2 − 1 A= i + {b\over 2} - 1 A = i + 2 b − 1
具体证明:论文
高维中,皮克定理和欧拉公式 V − E + F = 2 V - E + F = 2 V − E + F = 2 V , E , F V,E,F V , E , F
例题:poj 1265
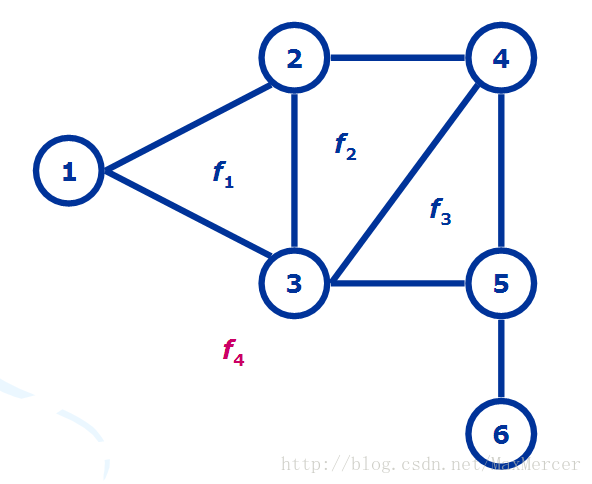
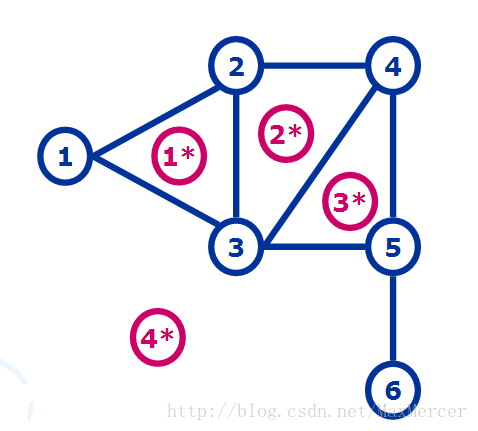
简单来说,平面图就是点和点之间有连边的图,如图:
而他的对偶图呢,就是把每个面抠出来,标上号,如果两个面之间有交点,那么就在对偶图中连一条边。
最后的对偶图建出来长这样:
对偶图的性质:平面图的最小割等价于它对偶图的最短路。(这好像就不属于计算几何的内容了 )。
至于平面图怎么转对偶图,啊我也不会,主要是网上的博客讲的太模糊了。
给你平面上一些点,让你求一个围墙能覆盖住这些点,且点到多边形的距离不能小于 L L L
求这个围墙的最小周长。 n , L ≤ 1 0 5 n,L\leq 10^5 n , L ≤ 1 0 5
首先,我们考虑一下当 L = 0 L = 0 L = 0
当 L ≠ 0 L \neq 0 L = 0
对于一个点距离小于 L L L L L L
我们把这个图画一下:
根据肉眼观察,要求的是最外面那一圈的周长,并且他可以分为两部分:原凸包上的边和若干个圆弧组成。
根据肉眼观察可得若干个圆弧可以拼接成一个圆。
证明:∠ A K L = 180 − ∠ C A I , ∠ I M O = 180 − ∠ A I G , ∠ P G N = 180 − ∠ F G I \angle AKL = 180 - \angle CAI,\angle IMO = 180-\angle AIG,\angle PGN = 180-\angle FGI ∠ A K L = 1 8 0 − ∠ C A I , ∠ I M O = 1 8 0 − ∠ A I G , ∠ P G N = 1 8 0 − ∠ F G I ∠ S F Q = 180 − ∠ E F G , ∠ U E R = 180 − ∠ C E F , ∠ J C T = 180 − ∠ A C E \angle SFQ= 180-\angle EFG, \angle UER = 180-\angle CEF,\angle JCT = 180-\angle ACE ∠ S F Q = 1 8 0 − ∠ E F G , ∠ U E R = 1 8 0 − ∠ C E F , ∠ J C T = 1 8 0 − ∠ A C E
外角相加等于 n ∗ 180 − ( n − 2 ) ∗ 180 = 360 n*180 - (n-2)*180 = 360 n ∗ 1 8 0 − ( n − 2 ) ∗ 1 8 0 = 3 6 0
所以我们最后的答案就是:凸包的周长加上一个圆的周长。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;const int N = 1e5 +10 ;const double pi = acos (-1.0 );const double eps = 1e-8 ;int n,r,top;double ans;struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }sta[N],p[N]; point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} point retate (point a,double alpha) {return point (a.x*cos (alpha)-a.y*sin (alpha),a.x*sin (alpha)+a.y*cos (alpha));}double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } bool comp (point a,point b) if (a.x == b.x) return a.y < b.y; return a.x < b.x; } int main () scanf ("%d%d" ,&n,&r); for (int i = 1 ; i <= n; i++) scanf ("%lf%lf" ,&p[i].x,&p[i].y); sort (p+1 ,p+n+1 ,comp); sta[++top] = p[1 ]; sta[++top] = p[2 ]; for (int i = 3 ; i <= n; i++) { while (top >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) < 0 ) top--; sta[++top] = p[i]; } int tmp = top; sta[++top] = p[n]; sta[++top] = p[n-1 ]; for (int i = n-2 ; i >= 1 ; i--) { while (top-tmp >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) < 0 ) top--; sta[++top] = p[i]; } for (int i = 1 ; i < top; i++) ans += dis (sta[i],sta[i+1 ]); printf ("%.0lf\n" ,ans+2 *pi*r); return 0 ; }
给你一些奇怪的形状的图形,求他们并在一起的图形的周长.
和上个题一样,答案可以分为两部分:原凸包上的边和若干段圆弧。
最后的答案为凸包周长加上一个圆的周长。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;const int N = 1e5 +10 ;const double pi = acos (-1.0 );const double eps = 1e-12 ;int n,cnt,top;double x,y,theta,a,b,r,ans;struct point { double x,y; point (double a = 0 , double b = 0 ){x = a, y = b;} }p[N],sta[N],w[5 ]; typedef point Vector;point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double b){return point (a.x*b,a.y*b);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double len (point a) return sqrt (a.x*a.x+a.y*a.y);}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)); } point retate (point a,double alpha) double coss = cos (alpha), sinn = sin (alpha); return point (a.x*coss-a.y*sinn,a.x*sinn+a.y*coss); } int dcmp (double x) if (fabs (x) < eps) return 0 ; if (x > 0 ) return 1 ; return -1 ; } bool comp (point a,point b) double m = Cro (a-p[1 ],b-p[1 ]); if (dcmp (m) > 0 ) return 1 ; if (dcmp (m) == 0 && dis (a,p[1 ]) < dis (b,p[1 ])) return 1 ; return 0 ; } int main () scanf ("%d" ,&n); scanf ("%lf%lf%lf" ,&b,&a,&r); a = a * 0.5 - r; b = b * 0.5 - r; for (int i = 1 ; i <= n; i++) { scanf ("%lf%lf%lf" ,&x,&y,&theta); w[1 ] = point (a,b); w[2 ] = point (a,-b); w[3 ] = point (-a,b); w[4 ] = point (-a,-b); p[++cnt] = retate (w[1 ],theta)+point (x,y); p[++cnt] = retate (w[2 ],theta)+point (x,y); p[++cnt] = retate (w[3 ],theta)+point (x,y); p[++cnt] = retate (w[4 ],theta)+point (x,y); } for (int i = 1 ; i <= cnt; i++) { if (dcmp (p[i].y-p[1 ].y) < 0 ) swap (p[i],p[1 ]); else if (dcmp (p[i].y-p[1 ].y) == 0 && dcmp (p[i].x-p[1 ].x) < 0 ) swap (p[i],p[1 ]); } sort (p+2 ,p+cnt+1 ,comp); sta[++top] = p[1 ]; sta[++top] = p[2 ]; for (int i = 3 ; i <= cnt; i++) { while (top >= 2 && Cro (sta[top]-sta[top-1 ],p[i]-sta[top-1 ]) <= 0 ) top--; sta[++top] = p[i]; } for (int i = 1 ; i < top; i++) ans += dis (sta[i],sta[i+1 ]); ans += dis (sta[top],sta[1 ]); ans += 2 * pi * r; printf ("%.2lf" ,ans); return 0 ; }
因为求的是最小的时间,考虑二分答案。
我们现在时间确定了,那么每个巫师能打死的小精灵的数量也是确定的。
考虑用网络流求解。
从源点向巫师连一条容量为巫师能打死的小精灵的数量,从每个巫师向他能打到的小精灵连一条容量为 1 1 1
再由每个小精灵向汇点连一条容量为 1 1 1
这样跑出来的最大流,就是我们最多能打死的精灵的数量。
现在想想,怎么求每个巫师能打死那个精灵。 n 3 n^3 n 3
因为每棵树的范围是一个圆,所以就相当于问你这个圆是否和巫师和小精灵的连线有交。
可以求出圆心到这条线段的最小距离,在和半径比较即可。
此外,还应该判断小精灵是否在巫师的攻击范围内,即点是否在圆内。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 101 102 103 104 105 106 107 108 109 110 111 112 113 114 115 116 117 118 119 120 121 122 123 124 125 126 127 128 129 130 131 132 133 #include <iostream> #include <cstdio> #include <algorithm> #include <queue> #include <cmath> #include <cstring> using namespace std;const double eps = 1e-8 ;const int inf = 1e7 +10 ;const int N = 2010 ;int n,m,k,u,v,s,t,res,tot = 1 ;int head[N],dep[N],tim[N],r[N],R[N];bool used[N][N];struct node { int to,net,w; }e[100010 ]; struct point { int x,y; point (){} point (int a,int b){x = a, y = b;} }c[N],p[N],tr[N]; typedef point Vector;point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}double dis_PS (point p,point A,point B) if (Dot (p-A,B-A) < 0 ) return dis (p,A); if (Dot (p-B,A-B) < 0 ) return dis (p,B); return abs (Cro (A-p,B-p))/dis (A,B); } void add (int x,int y,int w) e[++tot].to = y; e[tot].w = w; e[tot].net = head[x]; head[x] = tot; } bool bfs () queue<int > q; for (int i = 0 ; i <= t; i++) dep[i] = 0 ; q.push (s); dep[s] = 1 ; while (!q.empty ()) { int x = q.front (); q.pop (); for (int i = head[x]; i; i = e[i].net) { int to = e[i].to; if (e[i].w && !dep[to]) { dep[to] = dep[x] + 1 ; q.push (to); if (to == t) return 1 ; } } } return 0 ; } int dinic (int x,int flow) if (x == t || !flow) return flow; int rest = flow, val = 0 ; for (int i = head[x]; i && rest; i = e[i].net) { int to = e[i].to; if (!e[i].w || dep[to] != dep[x] + 1 ) continue ; val = dinic (to,min (rest,e[i].w)); if (val == 0 ) dep[to] = 0 ; e[i].w -= val; e[i^1 ].w += val; rest -= val; } return flow - rest; } bool judge (int mid) s = 0 , t = n+m+1 , tot = 1 , res = 0 ; memset (head,0 ,sizeof for (int i = 1 ; i <= n; i++) add (s,i,(mid/tim[i])+1 ), add (i,s,0 ); for (int i = 1 ; i <= m; i++) add (i+n,t,1 ), add (t,i+n,0 ); for (int i = 1 ; i <= n; i++) { for (int j = 1 ; j <= m; j++) { if (used[i][j]) add (i,j+n,1 ), add (j+n,i,0 ); } } int flow = 0 ; while (bfs ()) { while (flow = dinic (s,inf)) res += flow; } return res >= m; } int main () scanf ("%d%d%d" ,&n,&m,&k); for (int i = 1 ; i <= n; i++) scanf ("%d%d%d%d" ,&c[i].x,&c[i].y,&r[i],&tim[i]); for (int i = 1 ; i <= m; i++) scanf ("%d%d" ,&p[i].x,&p[i].y); for (int i = 1 ; i <= k; i++) scanf ("%d%d%d" ,&tr[i].x,&tr[i].y,&R[i]); for (int i = 1 ; i <= n; i++) { for (int j = 1 ; j <= m; j++) { int flag = 0 ; double tmp = dis (c[i],p[j]); if (tmp > r[i]) continue ; for (int l = 1 ; l <= k; l++) { double tmp = dis_PS (tr[l],c[i],p[j]); if (tmp <= R[l]) flag = 1 ; } if (flag == 0 ) used[i][j] = 1 ; } } int L = 0 , R = 500000 , ans = -1 ; while (L <= R) { int mid = (L + R)>>1 ; if (judge (mid)) { R = mid - 1 ; ans = mid; } else L = mid + 1 ; } printf ("%d\n" ,ans); return 0 ; }
考虑二分答案,转化为判定问题。
设抛物线的方程为: y = a x 2 + b x y = ax^2+bx y = a x 2 + b x
显然能通关一关当且仅当: y 1 ≤ a x 2 + b x ≤ y 2 y_1\leq ax^2+bx \leq y_2 y 1 ≤ a x 2 + b x ≤ y 2
化简一下: ${y_1\over x}\leq ax+b \leq {y_2\over x} $。
即: a x + b − y 1 x > 0 ax+b-{y_1\over x} > 0 a x + b − x y 1 > 0 a x + b − y 2 x < 0 ax+b-{y_2\over x} < 0 a x + b − x y 2 < 0
把 a , b a,b a , b x , y x,y x , y a , b a,b a , b
每次判定的时候,把 前 m i d mid m i d
剩下的就是你卡精度的问题了
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 101 102 103 104 105 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;#define double long double const double lim = 1e14 ;const double eps = 1e-14 ;const int N = 3e5 +10 ;int n,cnt,ans;double x,l,r;struct point { double x,y; point (){} point (double a,double b){x = a; y = b;} }sta[N]; typedef point Vector;struct line { int id; point x,y; double ang; line (){} line (point a,point b,int i) { x = a; y = b; ang = atan2 (b.y-a.y,b.x-a.x); id = i; } }L[N],q[N]; inline int read () int s = 0 ,w = 1 ; char ch = getchar (); while (ch < '0' || ch > '9' ){if (ch == '-' ) w = -1 ; ch = getchar ();} while (ch >= '0' && ch <= '9' ){s = s * 10 + ch - '0' ; ch = getchar ();} return s * w; } point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}bool OnRight (point p,line s) return Cro (p-s.x,s.y-s.x) > 0 ;}bool comp (line a,line b) if (a.ang == b.ang) return OnRight (b.x,a); return a.ang < b.ang; } int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } point Root_LL (line a,line b) Vector v = a.y-a.x, u = b.y-b.x, w = a.x-b.x; double t = Cro (w,u)/Cro (u,v); return a.x + v * t; } bool HPI (int mid) int l = 1 , r = 0 ; for (int i = 1 ; i <= cnt; i++) { if (dcmp (L[i].ang-L[i-1 ].ang) == 0 || L[i].id > mid) continue ; while (l < r && OnRight (sta[r-1 ],L[i])) r--; while (l < r && OnRight (sta[l],L[i])) l++; q[++r] = L[i]; if (l < r) sta[r-1 ] = Root_LL (q[r],q[r-1 ]); } while (l < r && OnRight (sta[r-1 ],q[l])) r--; while (l < r && OnRight (sta[l],q[r])) l++; if (r-l+1 <= 2 ) return 0 ; return 1 ; } int main () n = read (); point a = point (-lim,eps); point b = point (-eps,eps); point c = point (-eps,lim); point d = point (-lim,lim); L[++cnt] = line (a,b,0 ); L[++cnt] = line (b,c,0 ); L[++cnt] = line (c,d,0 ); L[++cnt] = line (d,a,0 ); for (int i = 1 ; i <= n; i++) { cin>>x>>l>>r; L[++cnt] = line (point (0 ,l/x),point (1 ,(l/x)-x),i); L[++cnt] = line (point (1 ,(r/x)-x),point (0 ,r/x),i); } sort (L+1 ,L+cnt+1 ,comp); int lx = 1 , rx = n; while (lx <= rx) { int mid = (lx + rx)>>1 ; if (HPI (mid)) { lx = mid + 1 ; ans = mid; } else rx = mid - 1 ; } printf ("%d\n" ,ans); return 0 ; }
solution
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;#define double long double const int N = 1e5 +10 ;const long long lim = 1e18 +10 ;const double eps = 1e-18 ;int n,m,cnt,num,l,r,ans[N];double k[N],w[N];struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }sta[N]; typedef point Vector;struct line { int id; point x,y; double ang; line (){} line (point a,point b,int i) { x = a; y = b; id = i; ang = atan2 (b.y-a.y,b.x-a.x); } }q[N],L[N]; int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}bool OnRight (point p,line a) return dcmp (Cro (p-a.x,a.y-a.x)-eps) >= 0 ;}point Root_LL (line a,line b) Vector v = a.y-a.x, u = b.y-b.x, w = a.x-b.x; double t = Cro (w,u)/Cro (u,v); return a.x + v * t; } bool comp (line a,line b) if (dcmp (a.ang-b.ang) == 0 ) return OnRight (b.x,a); return a.ang < b.ang; } int HPI () l = 1 , r = 1 ; q[1 ] = L[1 ]; for (int i = 2 ; i <= cnt; i++) { if (dcmp (L[i].ang-L[i-1 ].ang) == 0 ) continue ; while (l < r && OnRight (sta[r-1 ],L[i])) r--; while (l < r && OnRight (sta[l],L[i])) l++; q[++r] = L[i]; if (l < r) sta[r-1 ] = Root_LL (q[r],q[r-1 ]); } while (l < r && OnRight (sta[r-1 ],q[l])) r--; while (l < r && OnRight (sta[l],q[r])) l++; sta[r] = Root_LL (q[l],q[r]); if (r-l+1 == 2 ) return 0 ; return r-l+1 ; } int main () scanf ("%d" ,&n); for (int i = 1 ; i <= n; i++) cin>>w[i]; for (int i = 1 ; i <= n; i++) cin>>k[i]; point a = point (0 ,0 ); point b = point (lim,0 ); point c = point (lim,lim); point d = point (0 ,lim); L[++cnt] = line (a,b,0 ); L[++cnt] = line (b,c,0 ); L[++cnt] = line (c,d,0 ); L[++cnt] = line (d,a,0 ); for (int i = 1 ; i <= n; i++) { point a = point (0 ,w[i]); point b = point (1 ,k[i]+w[i]); L[++cnt] = line (a,b,i); } sort (L+1 ,L+cnt+1 ,comp); m = HPI (); for (int i = l; i <= r; i++) { for (int j = 1 ; j <= n; j++) { if (w[j] == w[q[i].id] && k[j] == k[q[i].id]) ans[++num] = j; } } sort (ans+1 ,ans+num+1 ); printf ("%d\n" ,num); for (int i = 1 ; i <= num; i++) printf ("%d " ,ans[i]); printf ("\n" ); return 0 ; }
给你一个平面直角坐标系,每个亲戚有其监视范围,问你小凸从 ( x , y ) (x,y) ( x , y )
不难想到:对于每个亲戚,向和他监视区域相临亲戚的连一条公共边,监视区域和边界相邻的向汇点连一条边,以一开始监视小凸的亲戚为源点,跑最短路,从源点到汇点的最短距离就是答案。
关键是怎么把图建出来。
对于两个点的情况,以两个点的中垂线为界,分成的两部分分别被两个人监控。如图:
黄色的区域就是 A A A
那么对于 n n n
所以,我们可以枚举每个亲戚,然后对他做一遍半平面交,最后队列中剩下的元素就是监视区域和他相连的亲戚。
在做半平面交的时候也要把四个边界加进去,如果队列中剩下的元素中有边界,那么就把这个点和汇点连一条边。
数据可能会有点不在这个矩形内部,特判掉即可。
最后每个亲戚的监视区域长成这个样子:
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 101 102 103 104 105 106 107 108 109 110 111 112 113 114 115 116 117 118 119 120 121 122 123 124 125 126 127 128 129 130 131 132 133 134 135 136 137 138 139 140 141 142 143 144 145 146 147 148 149 150 151 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> #include <queue> #include <cstring> using namespace std;const double eps = 1e-8 ;const int N = 1e5 +10 ;const int inf = 1e7 +10 ;int T,n,cnt,tot,s,l,r,d[N],head[N];bool vis[N],used[N];struct node { int to,net,w; }e[N]; struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }sta[N],p[N],st,en; typedef point Vector;struct line { int id; double ang; point x; Vector v; line (){} line (point a,Vector b,int i) { x = a; v = b; id = i; ang = atan2 (b.y,b.x); } }L[N],q[N]; int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} point retate (point a) {return point (-a.y,a.x);}double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}bool OnRight (point p,line a) return dcmp (Cro (p-a.x,a.v)) >= 0 ;}point Root_LL (line a,line b) Vector v = a.v, u = b.v, w = a.x-b.x; double t = Cro (w,u)/Cro (u,v); return a.x + v * t; } bool comp (line a,line b) if (dcmp (a.ang-b.ang) == 0 ) return OnRight (b.x,a); return a.ang < b.ang; } void add (int x,int y,int w) e[++tot].to = y; e[tot].w = w; e[tot].net = head[x]; head[x] = tot; } void HPI () sort (L+1 ,L+cnt+1 ,comp); l = 1 , r = 1 ; q[1 ] = L[1 ]; for (int i = 2 ; i <= cnt; i++) { if (dcmp (L[i].ang-L[i-1 ].ang) == 0 ) continue ; while (l < r && OnRight (sta[r-1 ],L[i])) r--; while (l < r && OnRight (sta[l],L[i])) l++; q[++r] = L[i]; if (l < r) sta[r-1 ] = Root_LL (q[r],q[r-1 ]); } while (l < r && OnRight (sta[r-1 ],q[l])) r--; while (l < r && OnRight (sta[l],q[r])) l++; sta[r] = Root_LL (q[l],q[r]); } void Dij () priority_queue<pair<int ,int > > Q; for (int i = 0 ; i <= n+2 ; i++) d[i] = inf, vis[i] = 0 ; d[s] = 0 ; Q.push (make_pair (0 ,s)); while (!Q.empty ()) { int t = Q.top ().second; Q.pop (); if (vis[t]) continue ; vis[t] = 1 ; for (int i = head[t]; i; i = e[i].net) { int to = e[i].to; if (d[to] > d[t] + e[i].w) { d[to] = d[t] + e[i].w; Q.push (make_pair (-d[to],to)); } } } } int main () scanf ("%d" ,&T); while (T--) { scanf ("%d" ,&n); tot = 0 ; memset (used,0 ,sizeof memset (head,0 ,sizeof scanf ("%lf%lf%lf%lf" ,&en.x,&en.y,&st.x,&st.y); for (int i = 1 ; i <= n; i++) { scanf ("%lf%lf" ,&p[i].x,&p[i].y); if (p[i].x > en.x || p[i].y > en.y) used[i] = 1 ; } if (n == 0 ) { printf ("%d\n" ,0 ); continue ; } double res = inf; for (int i = 1 ; i <= n; i++) { if (res > dis (p[i],st)) s = i; res = min (res,dis (p[i],st)); } for (int i = 1 ; i <= n; i++) { cnt = 0 ; if (used[i]) continue ; L[++cnt] = line (point (0 ,en.y),point (0 ,-1 ),n+1 ); L[++cnt] = line (point (en.x,0 ),point (0 ,1 ),n+1 ); L[++cnt] = line (point (0 ,0 ),point (1 ,0 ),n+1 ); L[++cnt] = line (en,point (-1 ,0 ),n+1 ); for (int j = 1 ; j <= n; j++) { if (j == i || used[j]) continue ; Vector v = retate (p[j]-p[i]); point o = point ((p[i].x+p[j].x)/2 ,(p[i].y+p[j].y)/2 ); L[++cnt] = line (o,v,j); } HPI (); for (int j = l; j <= r; j++) add (i,q[j].id,1 ); } Dij (); printf ("%d\n" ,d[n+1 ]); } return 0 ; }
由题意可得: S Δ P A 0 A 1 < S Δ P A i A i + 1 S_{\Delta PA_0A_1} < S_{\Delta PA_iA_{i+1}} S Δ P A 0 A 1 < S Δ P A i A i + 1
然后就开始暴力展开,推柿子。
A 0 A 1 ⃗ × A 0 P ⃗ < A i A i + 1 ⃗ × A i P ⃗ \vec {A_0A_1} \times \vec{A_0P} <\vec{A_iA_{i+1}} \times \vec {A_iP} A 0 A 1 × A 0 P < A i A i + 1 × A i P
设 $P = (x,y) $ , A i = ( x i , y i ) A_i = (x_i,y_i) A i = ( x i , y i )
( x 1 − x 0 , y 1 − y 0 ) × ( x − x 0 , y − y 0 ) < ( x i + 1 − x i , y i + 1 − y i ) × ( x − x i , y − y i ) (x_1-x_0,y_1-y_0) \times (x-x_0,y-y_0) < (x_{i+1}-x_i,y_{i+1}-y_i) \times (x-x_i,y-y_i) ( x 1 − x 0 , y 1 − y 0 ) × ( x − x 0 , y − y 0 ) < ( x i + 1 − x i , y i + 1 − y i ) × ( x − x i , y − y i )
( x 1 − x 0 ) ( y − y 0 ) − ( x − x 0 ) ( y 1 − y 0 ) < ( x i + 1 − x i ) ( y − y i ) − ( x − x i ) ( y i + 1 − y i ) (x_1-x_0)(y-y_0) - (x-x_0)(y_1-y_0) < (x_{i+1}-x_i)(y-y_i) - (x-x_i) (y_{i+1}-y_i) ( x 1 − x 0 ) ( y − y 0 ) − ( x − x 0 ) ( y 1 − y 0 ) < ( x i + 1 − x i ) ( y − y i ) − ( x − x i ) ( y i + 1 − y i )
x 1 y − x 1 y 0 − x 0 y + x 0 y 0 − ( x y 1 − x y 0 − x 0 y 1 + x 0 y 0 ) < x i + 1 y − x i + 1 y i − x i y + x i y i − ( x y i + 1 − x y i − x i y i + 1 + x i y i ) x_1y-x_1y_0-x_0y+x_0y_0 - (xy_1-xy_0-x_0y_1+x_0y_0) < x_{i+1}y - x_{i+1}y_i- x_iy + x_iy_i - (xy_{i+1} - xy_i - x_{i}y_{i+1} + x_iy_i) x 1 y − x 1 y 0 − x 0 y + x 0 y 0 − ( x y 1 − x y 0 − x 0 y 1 + x 0 y 0 ) < x i + 1 y − x i + 1 y i − x i y + x i y i − ( x y i + 1 − x y i − x i y i + 1 + x i y i )
$x_1y-x_1y_0-x_0y-xy_1+xy_0+x_0y_1 < x_{i+1}y-x_{i+1}y_i - x_iy - xy_{i+1} + xy_i + x_iy_{i+1} $
$(y_0-y_1) x + (x_1-x_0)y + x_0y_1 - x_1y_0 < (y_i-y_{i+1})x + (x_{i+1}-x_i)y + x_iy_{i+1} -x_{i+1}y_i $
( y 0 − y 1 − y i + y i + 1 ) x + ( x 1 − x 0 − x i + 1 + x i ) y + x 0 y 1 − x 1 y 0 + x i + 1 y i − x i y i + 1 < 0 (y_0-y_1-y_i+y_{i+1})x + (x_1-x_0-x_{i+1}+x_i)y + x_0y_1-x_1y_0 + x_{i+1}y_{i} - x_iy_{i+1} < 0 ( y 0 − y 1 − y i + y i + 1 ) x + ( x 1 − x 0 − x i + 1 + x i ) y + x 0 y 1 − x 1 y 0 + x i + 1 y i − x i y i + 1 < 0
( y 1 − y 0 − y i + 1 + y i ) x + ( x 0 − x 1 − x i + x i + 1 ) y + x 1 y 0 − x 0 y 1 + x i y i + 1 − x i + 1 y i > 0 (y_1-y_0-y_{i+1}+y_i) x + (x_0-x_1 - x_i+x_{i+1})y + x_1 y_0-x_0y_1 + x_{i}y_{i+1} - x_{i+1}y_{i} > 0 ( y 1 − y 0 − y i + 1 + y i ) x + ( x 0 − x 1 − x i + x i + 1 ) y + x 1 y 0 − x 0 y 1 + x i y i + 1 − x i + 1 y i > 0
那么合法的 p p p
最后的答案就是多边形面积除以半平面交区域的面积。
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 #include <iostream> #include <cstdio> #include <algorithm> #include <cmath> using namespace std;const int N = 1e5 +10 ;const double eps = 1e-8 ;int n,l,r,cnt,top;double ans1,ans2;struct point { double x,y; point (){} point (double a,double b){x = a, y = b;} }p[N],sta[N],Ans[N]; typedef point Vector;struct line { point x,v; double ang; line (){} line (point a,point b) { x = a; v = b; ang = atan2 (b.y,b.x); } }L[N],q[N]; int dcmp (double x) if (fabs (x) < eps) return 0 ; return x > 0 ? 1 : -1 ; } point operator + (point a,point b){return point (a.x+b.x,a.y+b.y);} point operator - (point a,point b){return point (a.x-b.x,a.y-b.y);} point operator * (point a,double k){return point (a.x*k,a.y*k);} double Dot (point a,point b) return a.x*b.x+a.y*b.y;}double Cro (point a,point b) return a.x*b.y-a.y*b.x;}double dis (point a,point b) return sqrt ((a.x-b.x)*(a.x-b.x) + (a.y-b.y)*(a.y-b.y));}bool OnRight (point p,line s) return dcmp (Cro (p-s.x,s.v)) >= 0 ;}point Root_LL (line a,line b) Vector v = a.v, u = b.v, w = a.x-b.x; double t = Cro (w,u) / Cro (u,v); return a.x + v * t; } bool comp (line a,line b) if (dcmp (a.ang-b.ang) == 0 ) return OnRight (b.x,a); return a.ang < b.ang; } void HPI () sort (L+1 ,L+cnt+1 ,comp); l = 1 , r = 1 , q[1 ] = L[1 ]; for (int i = 2 ; i <= cnt; i++) { if (dcmp (L[i].ang-L[i-1 ].ang) == 0 ) continue ; while (l < r && OnRight (sta[r-1 ],L[i])) r--; while (l < r && OnRight (sta[l],L[i])) l++; q[++r] = L[i]; if (l < r) sta[r-1 ] = Root_LL (q[r],q[r-1 ]); } while (l < r && OnRight (sta[r-1 ],q[l])) r--; while (l < r && OnRight (sta[l],q[r])) l++; sta[r] = Root_LL (q[l],q[r]); for (int i = l; i <= r; i++) Ans[++top] = sta[i]; } int main () scanf ("%d" ,&n); for (int i = 0 ; i < n; i++) scanf ("%lf%lf" ,&p[i].x,&p[i].y); for (int i = 1 ; i < n-1 ; i++) ans1 += Cro (p[i]-p[0 ],p[(i+1 ) % n]-p[0 ]); for (int i = 0 ; i < n; i++) L[++cnt] = line (p[i],p[(i+1 ) % n]-p[i]); for (int i = 1 ; i < n; i++) { int t = (i+1 ) % n; double A = p[0 ].y - p[1 ].y - p[i].y + p[t].y; double B = p[1 ].x - p[0 ].x - p[t].x + p[i].x; double C = p[0 ].x * p[1 ].y - p[1 ].x * p[0 ].y + p[t].x * p[i].y - p[i].x * p[t].y; if (dcmp (B) == 0 ) L[++cnt] = line (point (-C/A,0 ),point (-B,A)); else L[++cnt] = line (point (0 ,-C/B),point (-B,A)); } HPI (); for (int i = 2 ; i < top; i++) ans2 += Cro (Ans[i]-Ans[1 ],Ans[i+1 ]-Ans[1 ]); printf ("%.4lf\n" ,ans2/ans1); return 0 ; }